226. Invert Binary Tree

Given the root of a binary tree, invert the tree, and return its root.

Example 1:

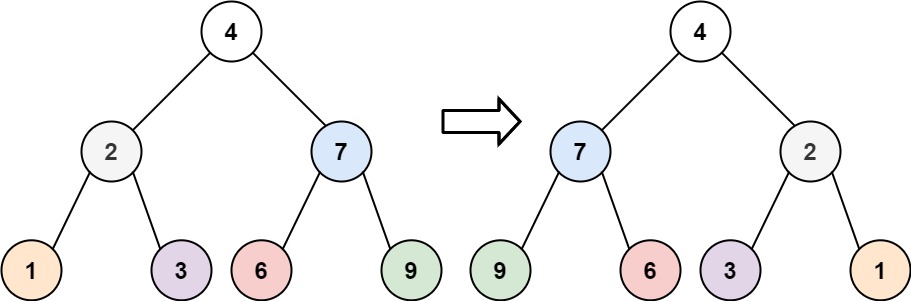
Input: root = [4,2,7,1,3,6,9]
Output: [4,7,2,9,6,3,1]

Example 2:

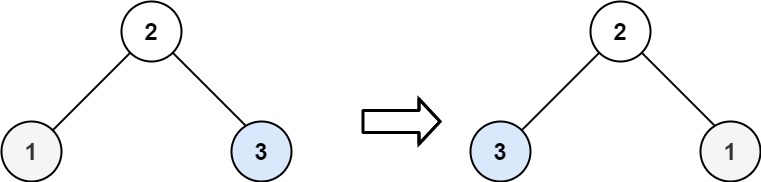
Input: root = [2,1,3]
Output: [2,3,1]

Example 3:

Input: root = []
Output: []

Constraints:

The number of nodes in the tree is in the range [0, 100].
-100 <= Node.val <= 100

題目意思

把一個 Binary tree 顛倒，很好理解，但是我沒有把 input 搞懂。
我一直以為 input 跟 output 會是一個 array，因為 example 就是寫成 array 的樣子。
但是其實 code 那邊註解有寫：

/**
 * Definition for a binary tree node.
 * function TreeNode(val, left, right) {
 *     this.val = (val===undefined ? 0 : val)
 *     this.left = (left===undefined ? null : left)
 *     this.right = (right===undefined ? null : right)
 * }
 */
/**
 * @param {TreeNode} root
 * @return {TreeNode}
 */

但是我沒有看懂，所以就直接用 array 來解題。

解題方向

看起來就是 tree 的每一個 tier 顛倒就好了，所以只要把每一個 tier 該做就事做好就好。

先算出有幾個 tier
每個 tier 的第一個 node 跟最後一個 node 對調，第二個 node 跟倒數第二個 node 對調，依此類推

var invertTree = function(root) {
  if (root.length === 0) return root

  let tierCount = 0
  while (true) {
    if (Math.pow(2, tierCount) === root.length + 1) break
    tierCount++
  }

  for (let tier = 0; tier < tierCount; tier++) {
    let left = Math.pow(2, tier)
    let right = Math.pow(2, tier) * 2 - 1
    while (left < right) {
      const temp = root[left - 1]
      root[left - 1] = root[right - 1]
      root[right - 1] = temp
      left++
      right--
    }
  }
  return root
};

但是這樣放上 leetcode 去跑，一直逾時，再簡單的 input 都逾時。
於是直接看解答才知道，原來 input 有 val, left, right 三個 attribute，分別是 node 的值，右邊的 child node，左邊的 child node。
原來註解寫的 TreeNode 是這個意思，懂了。
那這樣就很簡單了，而且已經偷看過答案所以就可以直接寫出來：

var invertTree = function(root) {
  if (!root) return root
  ;[root.left, root.right] = [root.right, root.left]
  if (root.left) invertTree(root.left)
  if (root.right) invertTree(root.right)
  return root
};

其他人的解法也都一樣，但我想再試看看不要用 recursive 來解看看：

var invertTree = function(root) {
  if (!root) return root
  let queue = [root]
  while (queue.length) {
    let currentNode = queue.pop() // DFS
    // let currentNode = queue.shift() // BFS
    ;[currentNode.left, currentNode.right] = [currentNode.right, currentNode.left]
    if (currentNode.left) queue.push(currentNode.left)
    if (currentNode.right) queue.push(currentNode.right)
  }
  return root
};

一開始沒什麼想法，但就是想到什麼就寫，結果發現一直往 tree 的下面做完之後，往上回來會有問題，因為沒辦法判斷 node 有沒有做過了。
所以又偷看了一下答案，原來要用一個 queue 來存放 node，這樣就明白了。
只要把 queue 裡面的 node 拿出來做，再把 child node 放進 queue 裡，只要 queue 裡面有東西，就一直做，直到 queue 做完。
比較有趣的是，看到有其他人的作法，我這樣的寫法是 DFS，只要把 pop() 改成 shift() 就變成 BFS 了。